RESEARCH DATA
|
Status: Completed, 2005 – 2010 Doctor: Ivo Stotz IBOIS EPFL Thesis director: Prof. Yves Weinand, architect and engineer, IBOIS Partners: Dr Eric Tosan, mathematician, LIRIS, Lyon 1, Iver Bailly Salins, mathematician DEA |
RESEARCH ABSTRACT
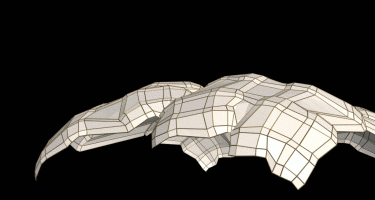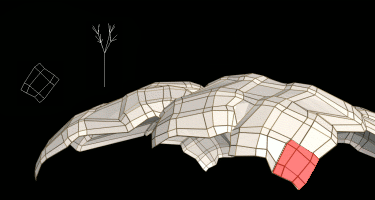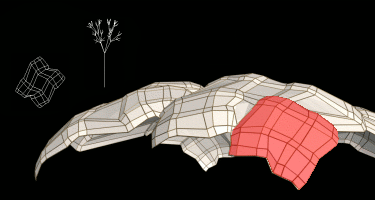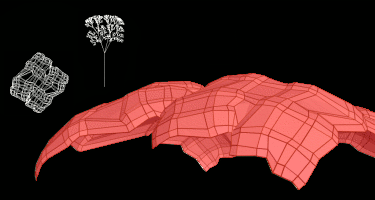
This thesis work is part of a broader research project that is entitled ‘Fractal Geometry and its Applications in the field of construction’. This interdisciplinary research gathers the domains of mathematics ( Dr E. Tosan LYON/LIRI ) and construction (Prof. Y. Weinand EPFL/IBOIS). The goal of the interdisciplinary work group is to research and to develop concrete applications of fractal geometry in the field of construction.
The project founds on the use a fractal model, based on iterative algorithms, which have been developed at the LIRIS for the creation of virtual images. The considered model is translated in order to create algorithms that are adapted to the problems of physical construction. This leads to the following speculation: Is it possible to use iterative algorithms – which produce fractal objects for virtual images – for the construction of materialized physical objects? Which are then the inherent specifications and advantages of this geometric modeling method?
The construction of fractals relies on BASRNSLEYS’ formalism, which uses iterative function systems. The formalism operates on a set of simple and comprehensible functions, which build – if applied iteratively – uncommon and complex objects. The way such objects are described is therefore limited to a few parameters. The obtained objects are always modeled of a discreet number of elements.
The discreet expression of the so modeled objects offers a bunch of advantages for the physical realization of complex shapes. The studied modeling method generates meshes that, in the one hand, are potentially interpretable by software for numerical simulation and, in the other hand, are ready made for integrated manufacturing. The proposed method not only allows the construction of fractal objects (selfsimilar objects, hollow fractal objects, growing structures, bursting objects) but also the construction of classic polynomial figures (Bezier, Spline, NURBS, etc.). In terms of architectural conception and production, the studied method opens the door to a new sculptural universe.
Further, the combination fractal geometry / wood / integrated manufacturing explores a new application range of the material wood in the field of construction. The so created structures will play different roles: Bearing structures and spatial separators as well as deco-, shading or acoustic panels.