
Probably the first historic report of plasmonic phenomena and arguably one of the most beautiful is the Lycurgus cup (British Museum, London, UK). Under ambient lighting, this fourth-century Roman chalice appears green, but when illuminated from the inside, the transmitted light shimmers bright red. Today we know that this fascinating behavior is due to nanoscopic gold and silver particles embedded in the glass. However, it took 1500 years and doubtless countless fantastic interpretations for a plausible explanation to emerge. In 1857, Faraday performed his experiments on metal colloidal solutions. He observed a change in color in dependence of the size and the material of the suspended particles. Just 51 years later, Gustav Mie presented an analytical treatment of the interaction of spherical particles with light. While Mie’s solution was able to explain the size and material dependency of the effects observed by Faraday, the physical processes were not yet fully understood.
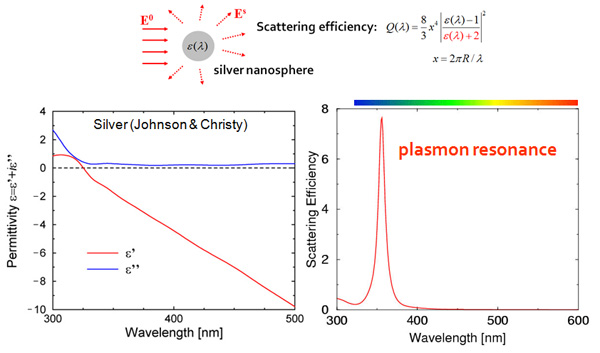
In electromagnetics, the response of a small particle to an external illumination is described by the permittivity ε(λ) of that particle, which depends on the illumination wavelength λ. For plasmonic metals (also called coinage metals since they are used to make coins) such as gold, silver, copper, aluminum, the permittivity is complex valued, i.e. has a real and imaginary parts, as illustrated for Silver in the previous figure: the real part ε’ in red is usually negative, while the imaginary part ε” in blue accounts for losses in the material; note how their values change with the wavelength. When the particle permittivity reaches the value ε’=-2, the denominator in the scattering efficiency formula vanishes and the amount of scattered light explodes. Since this phenomenon occurs for a specific wavelength (λ≈350nm in our example), light corresponding to this wavelength is strongly scattered, giving the particle its specific colors. One speaks of a plasmon resonance because the free electrons in the metal behave at this frequency like a solid state plasma in the charged metal ions.
Non-regular shape nanoparticles
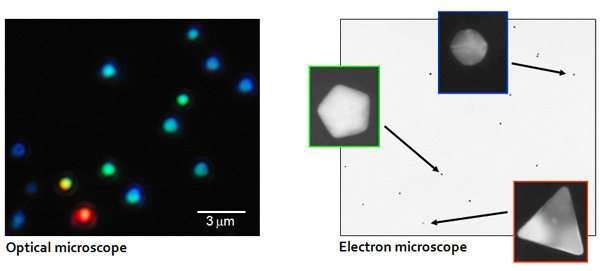
The above Mie theory explains the strong colours scattered by small silver nanoparticles in the blue. The experimental reality can however be quite different, as visible in the following dark field microscope image taken by Jack J. Mock from UCSD (data adapted from PNAS vol. 97, p. 996, 2000). While one would expect all silver particles to be blue, some appear yellow, green or even red! Resorting to the high magnification provided by electron microscope on the same sample indicates that the blue particles are spherical and well describes by Mie theory, while the non-regular shape particles shine different colours.
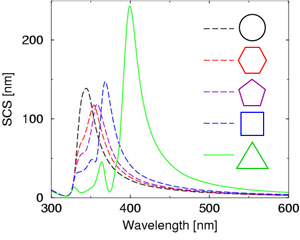
At the turn of the millennium, the NAM has devoted lots of efforts into understanding this surprising phenomenon and the relation between a metallic nanoparticle shape and its color. The next figure shows the scattering spectra computed for silver nanowires with different cross sections. When the symmetry of the section decreases (i.e. one goes from a circular section to a triangular one), many novel plasmonic modes appear at longer wavelengths. This explains that a low symmetry particle (i.e. a triangle) will have its plasmon resonance in the red, while a high symmetry one will have it in the blue. This understanding of light interactions with plasmonic nanoparticles of various shapes – as they appear in nature – has boosted research in the field!
The scattering cross section provides information on the color scattered by a particle in the far-field, i.e. at large distances from the particle. Having discovered that those colors depend on the particle shape, we moved on and investigated the optical near-field, very close to their surface. Here, we discovered that the electromagnetic field is much, much stronger in a realistic nanoparticle with an irregular shape, compared to what is predicted by Mie theory for spherical nanoparticles. This conceptual breakthrough provided a solid explanation for several interesting effects, such as surface-enhanced Raman scattering, where the near-field produced by a plasmonic nanostructure can enhance the vibration modes of a molecule deposited on that nanostructure.
Optical antennas
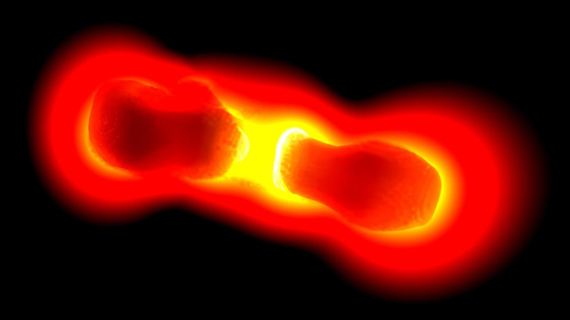
To increase the field enhancement further, one can use two plasmonic particles, as illustrated in the image above. The coupling between both nanostructures produces a dramatic enhancement of light. This concept is very similar to that of a dipole antenna, where two coupled metallic structures radiate very efficiently. With colleagues from Basel University, we introduced the concept of an optical antenna, which has become a flourishing field of research of itself.
Although these optical antennas rely on nanotechnology and work at optical wavelengths, they can still be investigated from an electrical engineering perspective, e.g. by computing optical field lines as shown in the image below.

At the NAM, we are using plasmonic antennas for a broad variety of applications, including enhanced fluorescence, Raman spectroscopy, plasmonic trapping, nonlinear optics, metasurfaces and biosensing.