Dislocations are the carriers of plastic deformation, and hence are the key defect for study in metals. It is also now well-established that plasticity is size-dependent, the origins of which must lie in the interactions among dislocations. Modeling of dislocation networks is usually at scales too large to execute in atomistic simulations so that continuum methods are necessary. In addition to atomistic studies of one or a few dislocations, as described elsewhere, we have been developing and applying continuum-level models.
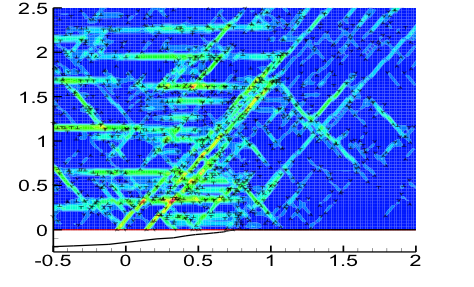
One major line of study has been “2d” and “2.5d” discrete dislocation (DD) dynamics, where the motion of individual dislocations, modeling as straight lines in plane strain geometries, under various imposed boundary conditions (tension, bending, fracture tests) are applied. We have made several crucial algorithmic improvements to existing methods, developed models that connect detailed DD input parameters to macroscopic flow, and applied the models to uncover new results. We have found that the yield strength is controlled by gradients in applied stress, leading to the new concept of “stress gradient plasticity” that has been supported by DD studies and explains a range of experiments on micron-scale samples. We have carefully studied fracture toughness in this class of materials, and found that the fracture toughness scales with the natural internal length scale of stress-gradient plasticity, providing an important basis for understanding fracture. We are currently extending the 2d DD method to include forest strengthening and work hardening in a natural manner within a 2d setting, leading to a so-called “2.5d DD” model.
The connection between atomistic dislocations and continuum dislocations is usually made through the application of elasticity theory and an approximate treatment of the dislocation core. It is important that continuum models properly represent the real atomistic dislocations. We have thus recently been investigating the properties of curved dislocations at the atomistic scale to measure the “line tension” associated with increased curvature of dislocations, and we are obtaining both quantitative values for particular systems as well as limitations to the application of continuum models.
Key publications:
- M. Wallin, W. A. Curtin, M. Ristinmaa, and A. Needleman, “Multiscale plasticity modeling: coupled discrete dislocation and continuum crystal plasticity”, J. Mech. Phys. Solids 56, 3167-3180 (2008).
- S. S. Chakravarthy and W. A. Curtin, “Stress-Gradient Plasticity”, Proc. Natl. Acad. Sci. 108, 15716-15720 (2011).
- S. Chakravarthy and W. A. Curtin, “Origin of length scale effects in fracture”, Phys. Rev. Lett. 105, Art. #115502 (2010).
- S. S. Chakravarthy and W. A. Curtin, “New algorithms for discrete dislocation modeling of fracture”, Mod. Sim. Matls. Sci. Eng. 19, Art. no. 045009 (2011).
Additional work:
- M. P. O’day and W. A. Curtin, “Superposition Method for Discrete Dislocation Plasticity”, J. Appl. Mech. 71, 805 (2004).
- M. P. O’day and W. A. Curtin, “Bimaterial Interface Fracture: a Discrete Dislocation Model”, J. Mech. Phys. Solids 53, 359 (2005).
- D. Olmsted, L.G. Hector, W.A. Curtin, and R.J. Clifton, “Atomistic simulations of dislocation mobility in Al, Ni, and Al/Mg alloys”, Mod. Sim. Matls. Sci. Eng. 13, 371 (2005).
- M. P. Dewald and W. A. Curtin, Multiscale Modeling of Dislocation/Grain-Boundary Interactions: I. Edge Dislocations Impinging on Sigma_11 (113) Tilt Boundary in Al, Mod. Sim. Matls. Sci. Eng. 15, S193-S215 (2007).
- M. Dewald and W. A. Curtin, Multiscale modeling of dislocation/grain-boundary interactions: III. 60 degrees dislocations impinging on Sigma 3, Sigma 9 and Sigma 11 tilt boundaries in Al”, Mod. Sim. Matls. Sci. Eng. 19, Art. no. 055002 (2011)
- M. P. Dewald and W. A. Curtin, “Multiscale Modeling of Dislocation/Grain-Boundary Interactions: II. Screw Dislocations Impinging on Tilt Boundaries in Al”, Phil. Mag. 87, 4615-4641 (2007).
- D. K. Ward, D. Farkas, J. Lian, W. A. Curtin, J. Wang, K-S Kim, and Y. Qi, “Engineering size-scaling of plastic deformation in Nanoscale asperities”, Proc. Natl. Acad. Sci. 24, 9580-9585 (2009).
- S. Chakravarty and W. A. Curtin, “Effect of source and obstacle strengths on yield stress: A discrete dislocation study”, J. Mech. Phys. Sol. 58, 625-635 (2010).
- T. Bhandakkar, A. C. Chng, W. A. Curtin, and H. J. Gao, “Dislocation shielding of a cohesive crack”, J. Mech. Phys. Sol. 58, 530-541 (2010).
- J. Song, W. A. Curtin, T. Bhandakkar, and H. J. Gao, “Dislocation Shielding and Crack Tip Decohesion at the Atomistic Scale”, Acta Mater. 58, 5933-5940 (2010).
- Y. Dong, T. Nogaret, and W. A. Curtin, “Scaling of Dislocation Strengthening by Multiple Obstacle Types”, Met. Trans. 41A, 1954-1960 (2010).
- S. Olarnirithinun, S. C. Chakravarthy, and W. A. Curtin, “Plasticity and Fracture in anisotropic plastic materials”, JMPS (2013)
- S. Chakravarthy and W. A. Curtin, “Stress Gradient Plasticity: Concept and Applications”, IUTAM (2013)
- B. Szajewski, S. Chakravarthy, and W. A. Curtin, “Operation of 3d Frank-Read Sources in a Stress Gradient”, Acta Mater. (2013)
- B. Szajewski and W. A. Curtin, Image forces in atomistic simulations of curved dislocations, submitted to MSMSE (2014).
- B. Szajewski, F. Pavia, Y. Dong, and W. A. Curtin, Line tension via atomistics, in preparation.
- S. Keralavarma and W. A. Curtin, Forest and Particle Strengthening in 2.5d Discrete Dislocation Simulations, in preparation.