Slender multi-scale porous structures are very common in industry and nature (cf. figure 1 for some example). When they interact with an incompressible Newtonian fluid flow, a physical understanding of the arising phenomenon is complex due to the presence of at least two length scales characterizing the fluid flow. Computer-based simulations of the first principles describing this phenomenon are extremely expensive for the same reason.
A valid alternative to a full-scale description of the physics at play consists of the development of models splitting the smallest scale from the largest one and including afterwards the feedback of the microscopic structure in the macroscopic model as a set of parameters that can be computed solving
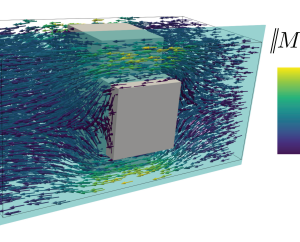
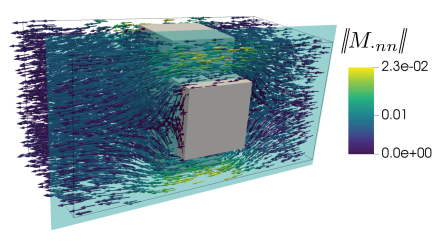
pore-scale problems (cf. figure 2). A homogenization based model to describe the flow of an incompressible newtonian fluid across thin microstructured surfaces has been developed in Zampogna & Gallaire(2020), assuming that the microstructure composing the whole, unbounded, skeleton is periodic. In the present project we extend the model already developed to include the macroscopic effects of the edges of the solid skeleton on the set of microscopic parameters present in the model. Simulations of fully resolved fluid flows across bounded structures will be carried out using Comsol Multiphysics and used to validate the new deriving model.

References:
Supervisor: Dr. Giuseppe Zampogna